I = U/R - i·ωσ
A·ds,
where U is the voltage difference between the two terminals of the solid conductor, and R is the DC resistance of the conductor.
AC magnetic analysis is the study of magnetic and electric fields arising from the application of an alternating (AC) current source, or an imposed alternating external field.
Variation of the field with respect to time is assumed to be sinusoidal. All field components and electric currents vary with time like
z = z0·cos(ωt + φz),
where z0 is a peak value of z, φz - its phase angle, and ω - the angular frequency.
Total current in a conductor can be considered as a combination of a source current produced by the external voltage and an eddy current induced by the oscillating magnetic field
j = j0 + jeddy.
If the field simulation is coupled with electric circuit, the branch equation for a conductor is:
I = U/R - i·ωσA·ds,
where U is the voltage difference between the two terminals of the solid conductor, and R is the DC resistance of the conductor.
The problem is formulated as a partial differential equation for the complex amplitude of vector magnetic potential A (B = curl A, B - magnetic flux density vector). The flux density is assumed to lie in the plane of model (xy or zr), while the vector of electric current density j and the vector potential A are orthogonal to it. Only jz and Az in planar or jθ and Aθ in axisymmetric case are not equal to zero. We will denote them simply j and A. The equation for planar case is
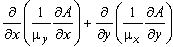- iωσA = -j0,
and for axisymmetric case is
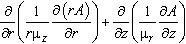- iωσA = -j0,
where electric conductivity σ and components of magnetic permeability tensor μx and μy (μz and μr) are constants within each block of the model. Source current density j0 is assumed to be constant within each model block in planar case and vary as 1/r in axisymmetric case.
Note. QuickField allows nonlinear materials with field-dependent permeability (ferromagnets) in AC magnetic formulation. This harmonic estimation makes use of a specially adjusted B-H curves providing energy conservancy over the AC period. This adjustment is performed automatically in the Curve Editor, it is recalculated after every change of the original curve made by the user. The curve editor for AC Magnetic problem presents both the user-defined and adjusted B-H curves.
The described formulation ignores displacement current density term ∂D/∂t in the Ampere's Law. Typically the displacement current density is not significant until the operating frequency approaches the MHz range.
Note. Permanent magnets cannot be simulated in a AC analysis. Since the entire field must vary sinusoidally, this would prevent permanent magnets from being simulated using the harmonic analysis as the permanent magnets supply a constant flux to the system.