I find QuickField is an invaluable tool in my study of crystal growth. I have been focusing on the atmospheric growth of ice crystals, for which there is a strong interplay between water vapor diffusion in the air and attachment kinetics at the crystal surface. The interesting physics is in the attachment kinetics, but to get at this one must first compensate for the effects of diffusion around the crystal. This is a non-trivial problem, but QuickField tackles it easily.

In the lab I grow simple hexagonal prism crystals, precisely measuring their growth rates under a range of well-controlled conditions. The crystal growth dynamics varies strongly with temperature and supersaturation, and I'm trying to better understand the surface physics that controls the growth. To interpret the measurements I need to model water vapor diffusion in the gas around the crystal, which is where I use QuickField. The heat diffusion module solves the relevant diffusion equation, and one need only cast the boundary conditions in the proper format. (For this purpose hexagonal prisms can be approximated as disks with negligible changes to the relevant physics.)

What I especially like about QuickField is that it is both powerful and easy to use. I don't have to be a full-time expert to use the software. Starting from scratch, in a matter of minutes I can try different parameters in order to better understand my experiments. The user interface is straightforward and easy to pick up, whether you're a new user or one who's not used the program in a while.

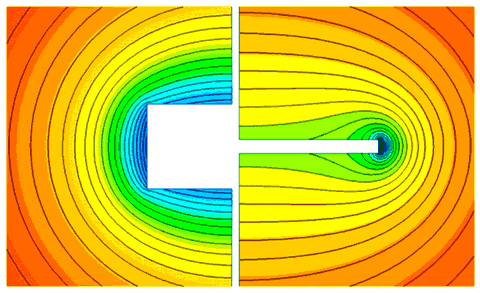
These plots show diffusion calculations around two growing ice crystals. The contours represent lines of constant supersaturation in the air around each crystal. The crystal on the left is a thick disk (cylindrically symmetric), and the right crystal is a thin disk. The central vertical line is the axis of symmetry for both crystals.

Kenneth G. Libbrecht
Professor of Physics and Physics Executive Officer
Caltech