Microstrip differential impedance

QuickField simulation example

This is an example of the microstrip line simulation, performed with QuickField software.

Problem Type
Plane-parallel problem of electrostatics and AC magnetics.

Geometry

Given
Relative permittivity of air ε = 1;
Relative permittivity of dielectric ε = 4.3.
Frequency f = 500 MHz.
Line length Lz = 5 inches

Task
Determine the differential impedance of the microstrip transmission line.

Solution
To calculate the differential impedance we should find the differential capacitance C_diff and the differential inductance L_diff.
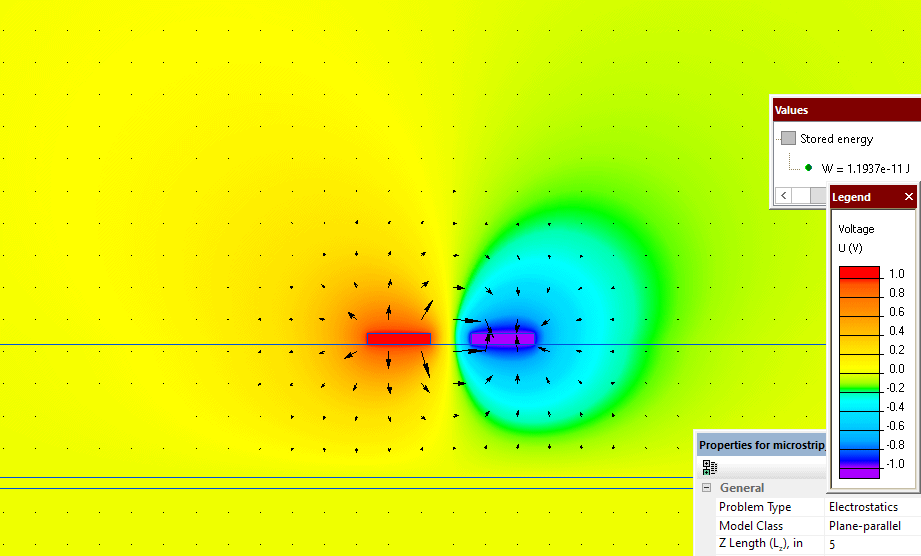
Capacitance is calculated in the electric problem. Positive potential is assigned to the left trace, negative potential is assigned to the right trace, zero potential is assigned to the ground plane. QuickField calculates the total stored electric field energy.
C_diff = 2*Energy / ( Potential difference )²

Inductance is calculated in the AC magnetic problem. In the left trace AC current flows in one direction. The return current flows in the right trace. Total current in ground plane is zero. QuickField calculates the total stored magnetic field energy.
L_diff = 2*Energy / |Current|²

Result
C_diff = 2*1.19e-11 / 2² = 6.0 pF (per 5" of line length)
L_diff = 2*4.15e-20 / (1e-6)² = 83 nH (per 5" of line length)
Impedance Z_diff = √L_diff/C_diff = 118 Ohm.

Voltage distribution in the microstrip line.

Current density distribution in the microstrip line.

• Video: Differential capacitance C_diff
  
• Video: Differential inductance L_diff
  
• Download simulation files (files may be viewed using any QuickField Edition).