Mutual inductance of coils

QuickField simulation example

Task
Find the dependence of mutual inductance of coaxial cylindrical coils upon the distance between them.

Experiment
Electro motive force (EMF) in the right coil is measured by ballistic galvanometer (at the switching on).

Problem type
Linear axisymmetrical problem of magnetostatics.

Geometry

Given
Relative magnetic permeability of air and copper coils μ= 1.
Current density in the left coil j=0.1 A/mm².
There is no current in the right coil, thus it has no affection to the field shape.

Solution
The field source is the lest coil. Due to the field symmetry only upper-right quarter aOb is defined. At the axes of symmetry the boundary conditions are set.
At the vertical axis of symmetry (line Ob) H_t=0. At the horizontal axis of symmetry Oa B_n=0. From B=rot A in the cylindrical coordinate system we have at the axis Oa A=const. Field fades at the infinity, so at the line Oa A=0 due to continuity of A.

Results
Flux density distribution around coils:

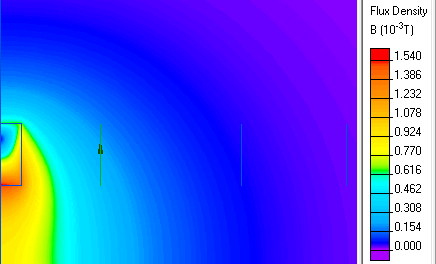
Mutual inductance M - relation of the flux connected with all turns of the right coil Ψ to the current in the left coil J (which is the origin of the flux).

L=Ψ / J
Ψ=Φ · w

Here w is number of turns of the right coil, Φ - flux across the right coil.

• View simulation report in PDF
• Download simulation files (files may be viewed using any QuickField Edition).