Halbach array generator voltage

QuickField simulation example

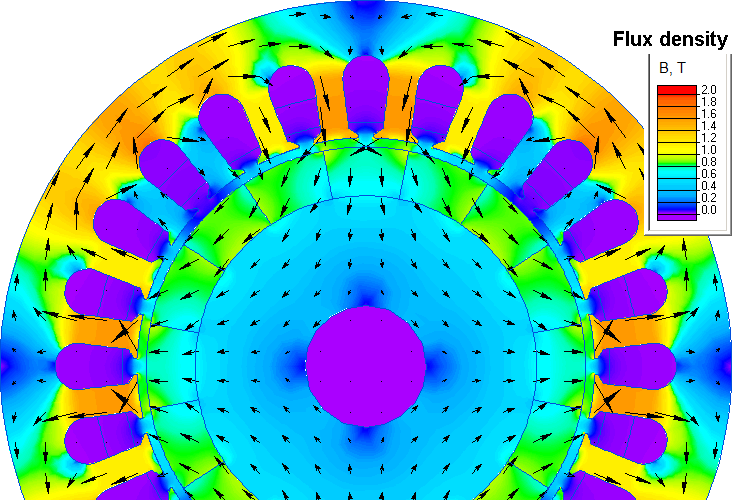
The excitation of the magnetic system on the rotor side is due to the Halbach assembly. The Halbach array is a special arrangement of permanent magnets that increases the magnetic field on one side of the assembly while cancelling it to near zero on the other side.

Problem type:
Plane-parallel problem of DC magnetics.

Geometry
Axial length is 50 mm.

Given
Stator phase coil number of turns w = 90,
Coercive force of permanent magnets 730 kA/m,
Rotor poles 2p = 4,
Synchronous rotation speed n = 750 rpm.

Task
Calculate the idle generator electromotive force (EMF).

Solution
In no-load there is no electric current in the winding, the output voltage is equal to the induced electromotive force.
First in QuickField we measure the flux linkage with half-coils Φ_A, Φ_a, Φ_B, Φ_b, Φ_C, Φ_c as a function of rotor angular position. We get this dependency automatically using the LabelMover parametric calculation utility. Then the phase winding flux linkage is calculated: Φ_Aa = Φ_A-Φ_a, Φ_Bb = Φ_B-Φ_b, Φ_Cc = Φ_C-Φ_c.
The electromotive force is calculated as the ratio of the flux linkage increment ΔΦ to the angle increment Δφ:
EMF = -w * dΦ/dt = -w * ΔΦ / (Δt), where time increment is related to the angle increment Δφ as: Δt = (Δφ/360) / (n/60).

Result
In LabelMover we set the angle increment to be Δφ = 1 degree. The time required to rotate the rotor by 1 degree is Δt = (1/360) / (750/60) = 2/9 ms.

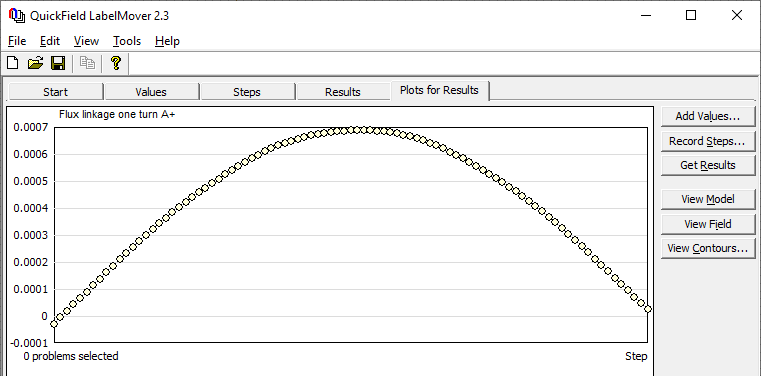
The caculated EMF is non-sinusoidal. Amplitude of the fundamental harmonic was computed with a harmonic analyzer tool: U₁ = 19 V.

Magnetic flux density distribution in the permanent-magnet generator

• Video: Halbach rotor generator. Watch on YouTube
  
• Download simulation files (files may be viewed using any QuickField Edition).