QuickField
A new approach to field modelling

Webinars

Virtual classroom

Tutorials

All videos

Main >> Video >> >> Turbine generator stator deformation

Turbine generator stator deformation

QuickField simulation example

During the design of a new turbine generator magnetic and mechanical vibration analysis should be performed. The magnetic analysis reveals that the stator yoke height of 330 mm would be sufficient - the magnetic flux density in the yoke is below 1.9 T. But the mechanical analysis indicates that deformations of the stator caused by the magnetic pull exceed the maximum vibration magnitude specified in IEC 60034-14*.

Problem Type
Plane-parallel multiphysics problem of DC magnetics coupled to Stress analysis.

Geometry
Axial length is 5400 mm.
Turbine generator stator vibrations Calculate the stator deformation Stator yoke Rotor A Z B X C Y Ø1740 mm 330 mm 1295 mm 1075 mm

Given
Steel Young's modulus E = 200 GPA, Poisson's ratio ν = 0.35.
Stator winding current density 2 A/mm² (r.m.s.), frequency 50 Hz.
Field winding current density 1 A/mm² (direct current).
Power angle 30°.
The B-H curve for the stator tooth zone:

The B-H curve for the stator yoke and the rotor:

Task
Calculate the stator deformations root mean square value.

Solution
The deformations are caused by the magnetic pull. So first, we simulate a magnetic problem. The rotor and stator rotate at a synchronous speed, so if we neglect changes in the mutual positions of the rotor and stator teeth, the magnetic force magnitude does not change in time, only the force direction varies. We can choose any moment of time, specify momentary values of the currents, and simulate the DC magnetic problem to calculate the magnetic field distribution and forces.
AC currents have the following momentary values: phase A 2e6*√2*cos(0), phase B 2e6*√2*cos(120), and phase C 2e6*√2*cos(240).

Results
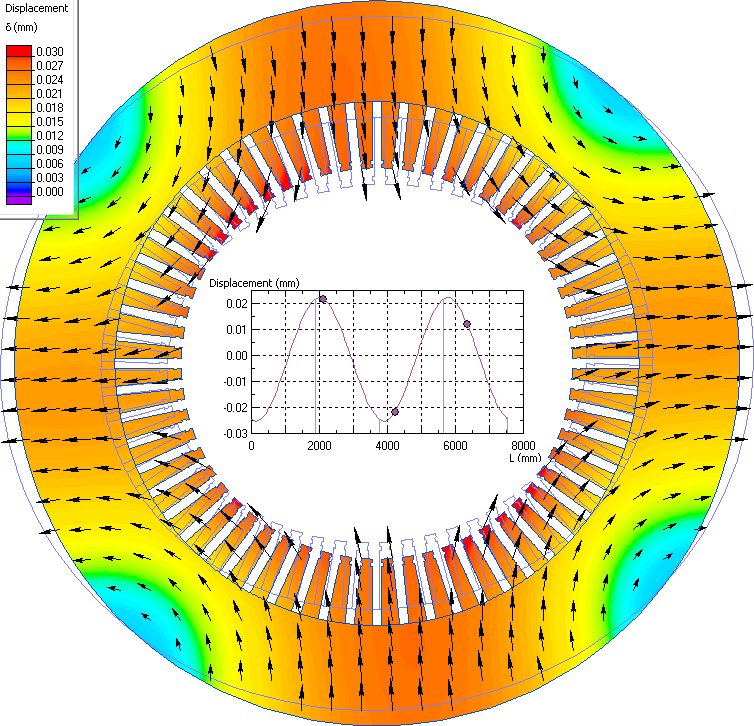
Magnetic flux density distribution in the turbine generator:
Turbine generator magnetic flux

The deformation double magnitude = xmax - xmin = 22.2 - (-25.3) = 47.5 μm exceeds the limits of the vibration magnitude 45 μm defined by IEC 60034-14. The stator diameter should be increased to make it stiffer.
Turbine generator vibrations

Reference
* IEC 60034-14 Rotating electrical machines - Part 14: Mechanical vibration of certain machines with shaft heights 56 mm and higher - Measurement, evaluation and limits of vibration severity.